26 March 2015

During the freezing of water, the initially unordered molecules start to form an ordered crystal, namely ice. During this phase transition, they rearrange from an unordered into a more ordered state. This setting naturally poses one important question: How long does this phase transition take, i.e. how long does it take for each molecule to find its place in the crystal? The answer to this and similar questions has important consequences in e.g. metallurgy, since the size of the resulting crystal grains plays a major role in determining the elasticity or brittleness of steel. While these questions have been studied extensively for classical systems, they are relatively unexplored in the context of quantum systems. By using ultracold atoms in optical lattices, a team led by Ulrich Schneider and Immanuel Bloch at the Max Planck Institute of Quantum Optics, the Ludwig-Maximilians-Universität Munich, the Freie Universität Berlin, and the Consejo Superior de Investigaciones Científicas in Madrid have succeeded in measuring the emergence of order in a clean and well controlled experiment. Their results reveal deficiencies in current theoretical models and have been published this week in the „Proceedings of the National Academy of Sciences“.

The essential question for any phase transition is: How does it actually happen, i.e. how does the system evolve from one phase to another? Especially for quantum systems there is typically no easy answer available, since the dynamics of these systems are typically much more complex than the phases themselves. In addition, there is an effect called critical-slowing-down, which means that the reaction time of the system strongly increases on approaching the phase transition point and diverges at this point. As a consequence, the system can never evolve completely “smooth” from an unordered into an ordered phase. How fast the correlations characterizing the ordered phase can emerge and spread, is therefore a central problem in the physics of phase transitions. Intuitively, one can imagine a collection of small arrows that initially point in random directions. Beyond the phase transition, each arrow now wants to point in the same direction as its neighbours. So, ultimately, all arrows want to be aligned but which direction will be chosen? Since at first all directions are equivalent, the arrows have to spontaneously break this symmetry, meaning they have to agree on one particular orientation. How fast can this process of ordering happen?

The emergence of ordered phases has been studied theoretically already for some time. Now for the first time the scientists were able to investigate this process in a clean and precisely controlled synthetic many-body system: This system is formed by up to a hundred thousand atoms that have been cooled deep into the quantum regime and then were localized to individual lattice site in a so-called optical lattice, that is a crystal of light formed by overlapping and interfering laser beams. In this Mott insulator there exist no correlations between lattice sites. By now increasing the coupling between the lattice sites—the tunneling rate of particles—a quantum phase transition into another phase can be induced. In this new, superfluid phase, the particles are free to move through the lattice. Since all atoms move in a coherent fashion—they will ultimately all be part of a single wave function, a Bose-Einstein condensate—this results in long-range correlations, even between very distant lattice sites. In their experiment, the experimental team in Munich could now for the first time observe this emergence of correlations quantitatively and study its time dependence. They could compare their results to theoretical models and show that these models are too simple to describe realistic situations and will need to be extended by novel and not yet known contributions.

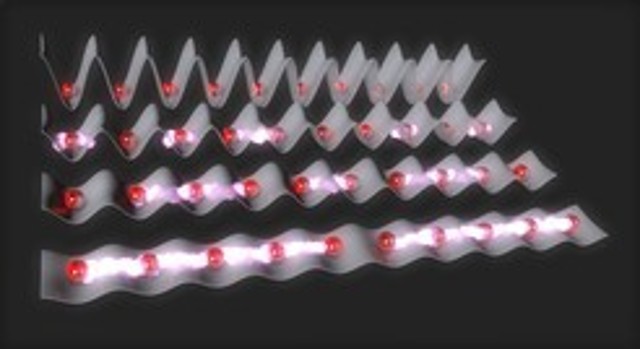
While the atoms are strongly localized in the initial state (upmost row) in the deep lattice, correlations develop during the crossing of the phase transition induced by lowering the lattice depth.
In the final weak lattice (lowest row), the correlations would in principle span the whole lattice, if the phase transition could be crossed infinitely slow. In reality, however, the finite ramp speed limits the correlation length to a finite value.

In the one-dimensional case, that is a string of lattice sites, the experimental results could be compared to exact numerical calculations performed on classical super-computers by the team around Jens Eisert from the Freie Universität Berlin. This comparison constitutes an independent check of the experiment, which it passed with flying colours. Motivated by this success, the experiment was then extended to two- and three dimensional situations, where no numerical calculations are feasible. The obtained results can now be used to test and benchmark novel theoretical concepts and thereby advance our fundamental knowledge and understanding of the many-body dynamics in quantum systems.

The present experiment can be viewed as a Quantum Simulator: By performing experiments on well-controlled model systems we can learn about the behaviour of complex systems, which are often challenging to directly study experimentally and impossible to simulate numerically. By providing much-needed benchmark data, Quantum Simulators will help to fundamentally advance our knowledge about such systems.
Original source [ here]
Dr. Ulrich Schneider

LMU München, Faculty of physics
Schellingstr. 4, 80799 Munich, Germany
Phone: +49 (0)89 / 2180 -6129
Prof. Dr. Immanuel Bloch

Chair of Quantum Optics, LMU München Schellingstr. 4, 80799 Munich
Director at Max Planck Institute of Quantum Optics
Hans-Kopfermann-Straße 1
85748 Garching, Germany
Phone: +49 (0)89 / 32 905 -138
Dr. Olivia Meyer-Streng Press & Public Relations Max Planck Institute of Quantum Optics, Garching, Germany