21 January 2015

Topology, a branch of mathematics classifying geometric objects, has been exploited by physicists to predict and describe unusual quantum phases: the topological states of matter. These intriguing phases, generally accessible at very low temperature, exhibit unique conductivity properties which are particularly robust against external perturbations, suggesting promising technological applications. The great stability of topological states relies on a set of magic integers, the so-called Chern numbers, which remain immune to defects and deformations. For the first time, an international team of scientists succeeded to measure the topological Chern number in a non-electronic system with high precision. The experiments were carried out with ultracold bosonic atoms controlled by lasers, in the group of Professor Immanuel Bloch (Ludwig-Maximilians-Universität Munich and Max Planck Institute of Quantum Optics, Garching) in collaboration with Nathan Goldman and Sylvain Nascimbène from the Collège de France and Nigel Cooper from Cambridge University. The result has been recently published in Nature Physics.

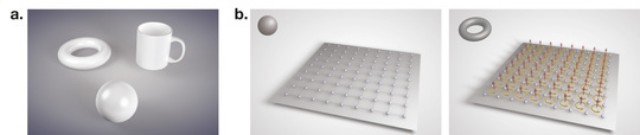
Matter forms remarkable phases when it is immersed in extreme environments, such as strong magnetic fields and low temperature. Under these conditions, materials can reach unusual regimes where their electrical properties present universal and exotic behaviours, e.g. dissipationless currents and quantized electrical resistance. This physical framework sets the stage for new phases of matter, the topological states, which are described by magic (topological) integers. They are mathematical numbers used to classify geometric objects [e.g. the number of holes in a surface, Fig. 1a], and which remain immune to deformations. The outstanding fact that quantum states of matter can be associated with topological numbers guarantees the robustness of their unique electrical properties against perturbations. This suggests numerous promising technological applications, e.g. in spintronics and quantum computation, hence motivating the search for novel topological states of matter in laboratories.

Topological states were discovered in the context of the quantum Hall effect, i.e. through studies of the electrical resistance in materials subjected to strong magnetic fields. After reaching sufficiently low temperatures, the measured resistance was found to form robust plateaus when varying the magnetic field, a behaviour which was shown to be independent of the sample. Surprisingly, this universal physical property - the quantum Hall effect celebrated by the Nobel prize in 1985 - appeared to be rooted in topology: each resistance plateau is dictated by a topological number, the Chern number. "The beauty of this result relies in the fact that these magic mathematical numbers appear as intrinsic properties of the electrons moving in the material; it is intriguing that these abstract numbers actually lead to extraordinary observable phenomena", says theorist Nathan Goldman.

An interesting route for the search of topological phases of matter is offered by synthetic materials, which consist of ultracold atomic gases controlled by light. In these highly versatile experiments, neutral atoms are trapped in a periodic landscape created by standing waves of lasers. Cold atoms moving in these optical lattices have proven to be very well suited to mimic the dynamics of electrons propagating in real materials. However, in contrast to electrons, cold atoms are charge neutral; hence, they do not exhibit the Hall effect in the presence of a magnetic field. To overcome this limitation, new experimental techniques were developed in Munich in order to engineer effective magnetic fields for neutral atoms. In such arrangements, cold atoms behave as charged particles subjected to strong magnetic fields, offering a new platform to study the Hall effect and topological phases in a highly controllable and clean environment.

The optical-lattice setup realized in the Munich experiment has been specifically tailored so as to exhibit topological properties (Fig. 1b). Indeed, when inducing an effective magnetic field in the lattice, the atomic gas is characterized by a non-zero topological Chern number ?ch = 1. Nathan Goldman explains: "In this configuration, and in direct analogy with the electric Hall effect, the atomic cloud is expected to experience a characteristic transverse motion in response to an applied force. Moreover, our theory predicts that this transverse drift should be directly proportional to the topological Chern number (?ch = 1)". The experimentalists applied a force to their optical-lattice setup and analyzed such a displacement by taking snap-shots of the cloud. From this sequence of images, they determined an experimental value for the Chern number ?exp = 0.99(5) in excellent agreement with theory. This result constitutes the first Chern-number measurement in a non-electronic system. In contrast to electronic measurements, which are based on currents flowing along the edges of the sample, the Munich Chern-number measurement directly probes the topological nature of the bulk.

These measurements constitute an important step towards the realization and detection of topological states with ultracold atoms. Including interactions between the atoms could generate novel and exciting phases, such as the much sought after fractional Chern insulators. [N.G. and M.A.] Prof. Dr. Immanuel Bloch
Chair of Quantum Optics, LMU München
Schellingstr. 4, 80799 Munich
Director at Max Planck Institute of Quantum Optics
Hans-Kopfermann-Straße 1 85748 Garching, Germany
Phone: +49 (0)89 / 32 905 -138
E-Mail: immanuel.bloch@...
Prof. Dr. Nathan Goldman
Collège de France, Laboratoire Kastler Brossel
11, place Marcelin Berthelot 75005 Paris, France,
and Center for Nonlinear Phenomena and Complex Systems,
Université Libre de Bruxelles, CP 231, Campus Plaine,
B-1050 Brussels, Belgium
Phone: +32 2 6505797
E-mail: nathan.goldman@lkb.ens.fr und ngoldman@ulb.ac.be
M. Sc. Monika Aidelsburger
LMU München, Faculty of physics
Schellingstr. 4, 80799 Munich, Germany
Phone: +49 (0)89 / 2180 -6119
E-mail: monika.aidelsburger@physik.uni-münchen.de
Dr. Olivia Meyer-Streng Press & Public Relations Max Planck Institute of Quantum Optics, Garching, Germany Phone: +49 (0)89 / 32 905 -213 Original Source